Describe the End Behavior of a Function

As x -00 fx 4 As x 00 fx -3. The graph of the function starts high and ends high.

How To Determine The End Behavior Of A Polynomial Function Polynomial Functions Polynomials Absolute Value Equations

Mar 15 2018 The end behavior of cubic functions or any function with an overall odd degree go in opposite directions.

. The end behavior of a function eqf x eq refers to how the function behaves when the variable eqx eq increases or decreases without bound. As x- - f x - As x- f x - - Detailed. Describe the end behavior of the graph of the function f x5 4x8.

As x f x. Experts are tested by Chegg as specialists in their subject area. Function End Behavior - 18 images - eritia cadiz 2021 all you need to know before you go with photos ex end long run behavior of exponential functions youtube end behavior and characteristics of functions youtube rational functions limits 1.

As x f x. Because the power of the leading term is the highest that term will grow significantly faster than the other terms as x gets very large or very small so its behavior will dominate the graph. If the values of a variablexincrease without bound then we writex and if the values ofxdecrease without bound then we writex.

Solved by verified expert. As x f x. If the value of the function is positive place it in Quadrant II.

X00 1 X-00 lim f x Simplify your answer lim f x Simplify your answer X--o0. Who are the experts. This problem has been solved.

Describe the end behavior of the graph of the function. Yes Advertisement Answer Expert Verified 47 5 14 danielmaduroh Right answer. How do you describe the end behavior of a cubic function.

Describe the end behavior of the function. The graph of the function starts low and ends high. We review their content and use your feedback to keep the quality high.

The graph of the function starts high and ends low. Can be rearranged to. The graph of the function starts low and ends low.

As you can see if x increases without bound then f x take values from the straight line but those values become more and more negative. We can use words or symbols to describe end behavior. Describe the end behavior of the function f x 4x4 4x5 16 by finding lim f x and lim f x.

As x f x. For example lim x 1 x 0 and lim x 1 x. For example in case of y f x.

F x anxn an1xn1 a1xa0 f x a n x n a n 1 x n 1. Cubic functions are functions with a degree of 3 hence cubic which is odd. To determine its end behavior look at the leading term of the polynomial function.

The behavior of the graph of a function as the input values get very small displaystyle xto -infty x and get very large displaystyle xto infty x is referred to as the end behavior of the function. As we have already learned the behavior of a graph of a polynomial function of the form. The end behavior of a function is a way of classifying what happens when x gets close to infinity or the right side of the graph and what happens when x goes towards negative infinity or the left.

As x -00 fx -4. Describe the end behavior of fx -5x 4 - 2x 2 8. 7 Steps to Graph a Polynomial.

The end behavior of a function is the behavior of the graph of the function f x as x approaches positive infinity or negative infinity. The table below shows the end behavior of power functions in the form. Which describes the end behavior of the function.

As x -20 fx -00 As x 20 f x -00. -As x approaches -6 from the right. This means that the sign of the leading coefficient is sufficient to predict the end behavior of the function.

In other words the end behavior of a function describes the trend of the graph if we look to the right end of the -axis as approaches and to the left end of the -axis as approaches. Endbehaviorfxlnx-5 endbehaviorfxfrac1x2 endbehavioryfracxx2-6x8 endbehaviorfxsqrtx3. As x f x.

A periodic function is basically a function that repeats after certain gap like waves. The end behavior of a polynomial function describes how the graph behaves as x x approaches. Fx 2x² x² A.

4 оооо As x 0 f 2 0. As x -0f2 As 27 00 fx 0. F x cos x and f x sin x are both periodic since their graph is wavelike and it repeats.

End Behavior of a Function. Will either ultimately rise or fall as x increases without bound and will either rise or fall as x decreases without bound. Where P is a nonzero constant commonly referred to as the fundamental period.

The end behavior of a function f describes the behavior of the graph of the function at the ends of the x-axis approaching infinity. A 1 x a 0. If the value of the function is negative place it in Quadrant III.

Do you have the answer to the whole test. Precalculus Polynomial Functions of Higher Degree End Behavior 1 Answer Aru R. For example the cosine and sine functions ie.

As x f x. The behavior of a function fxasxincreases without bound or decreases without bound is sometimes called theend behaviorof the function. This is determined by the degree and the leading coefficient of a polynomial function.

WILL GIVE BRAINLIEST Describe the end behavior of the following function. All tutors are evaluated by Course Hero as an expert in their subject area. As x f x.

In other words the end behavior describes the. The end behavior of a function is equal to its horizontal asymptotes slantoblique asymptotes or the quotient found when long dividing the polynomials. The end behavior of a function describes the behavior of the graph of the function at the ends of the -axis.

This is true because of the graph. For any polynomial the end behavior of the polynomial will match the end behavior of the term of. Without graphing describe the end behavior of the graph of the function.

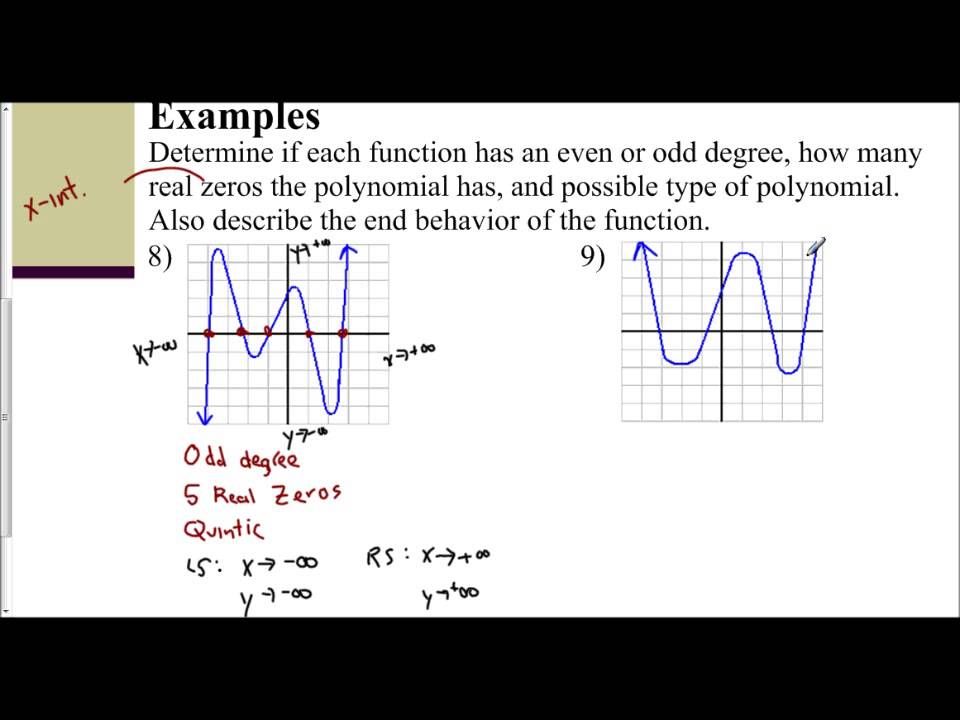
Lesson 6 3 Identifying Even Odd Degree Functions Zeros End Behavior Lesson Behavior Polynomial Functions

End Behavior Of A Polynomial Function A Graphing Calculator Investigation Polynomial Functions Polynomials Quadratics

Graphing And Finding Roots Of Polynomial Functions Math Hints Teaching Algebra School Algebra College Algebra

Graphing And Finding Roots Of Polynomial Functions She Loves Math Polynomials Polynomial Graph Polynomial Functions